Physiology I
Section 2
Excitable Cells
Resting Membrane Potential

Suggested Reading:  Guyton Chapter 5

Key Words

Steady State:  

Equilibrium:  

Electro-Neutrality:  Everywhere except adjacent to the surfaces of the cell membrane itself, the negative and positive charges are equal.  Guyton page 59

Nernst Equation:  +/- 60 log (concentration in / concentration out)  
Nernst potential= the potential level across the membrane that prevents net diffusion of an ion in either direction through the membrane

Membrane Potential:  Electrical potentials that exist across a cell membrane .   Caused by diffusion:  membrane permeable to K⁺ only because of large K⁺ concentration inside cell=strong force  for K+ to move out (By diffusion).  As they do they carry + charges to the outside, thus creating a state of electropositivity outside and electro negativity inside (because of the negative anions that remain behind and do not diffuse out with the K+).  This new potential (diffusion + outside, negative inside repels the positive charged K+ ions that are diffusing outward back into the cell.  Within a millisecond the potential change becomes great enough to block further net diffusion to the exterior despite the increase K+ concentration gradient.  This potential diffusion is approximately 94mV with negativity inside the membrane.  SO....A concentration diffusion of ions across a selectivity permeable membrane can cause the creation of a membrane potential.

Equilibrium Potential:  The point in a membrane potential where influx and efflux of  specific ion are equal.  It is defined for each ion by the Nernst Equation.

Resistance:  (R) Any Force that impedes the flow of current.  It is the inverse of conductance.

Conductance:  Any force that eases the flow of current.  It is the inverse of resistance.  

Voltage:  (E or V) The separation of charges maintained through the expenditure of energy or the driving force that moves charged particles.

Current:  (I) The passing of elections along a conduit or charges moving from one place to another.

Ohm's Law:  I=V/R  or  V=IR

Electro-Chemical Gradient:  Gradient brought about by difference of charges as well as difference in concentrations.

Goldman Equation:  Gives the calculated membrane potential on the inside of the membrane when 2 univalent positive ions, Na+ and K+ and one univalent negative ion Cl- are involved.

Semi-Permeable Membrane:  A membrane that allows passage of water but not substances in solution.

Learning Objectives

Discuss Ohm's Law, and the properties of Voltage, Current, and Resistance.  Use the hydraulic/fluid analogy if you wish.:  Ohm's Law= Current (I)=V/R

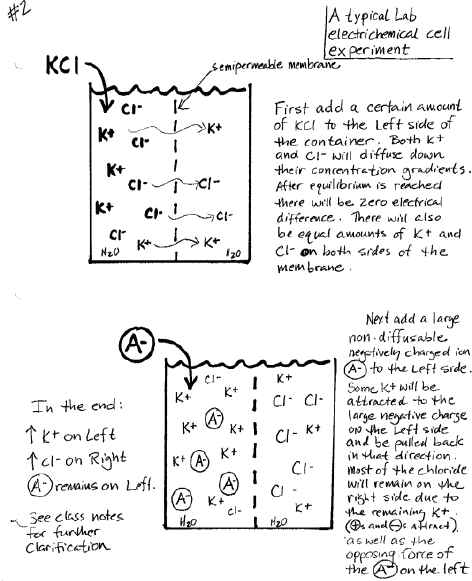
Describe the transient movements of all ions and the final steady-state conditions in a typical laboratory electrochemical cell experiment:  

Define and discuss "equilibrium potential" or "Nernst Potential", and give approximate values of the Nernst potentials for Na+, K+, and Cl- in typical nerve and muscle cells:  The potential level across the membrane that prevents net diffusion of an ion in either direction through the membrane is called the Nernst potential for that ion. The magnitude of this potential is determined by the ratio of the ion concentrations on the two sides of the membrane.  The greater this ratio, the greater the tendency for the ions to diffuse in one direction, and therefore the greater is the Nernst potential.  

Discuss the role of non-diffusible intracellular ions in the production and maintenance of the resting membrane potential:  Their role is simple to explain:  Because of the large potassium concentration gradient from the inside toward the outside, there is a strong tendency for K+ ions to move (diffuse) outward.  As they do so, they carry positive charges to the outside, creating a state of electropositivity outside the membrane and electronegativity on the inside... BECAUSE OF THE NEGATIVE ANIONS THAT REMAIN BEHIND AND DO NOT DIFFUSE OUTWARD along with K+.  This new potential difference (- in, + out) repels the K+ ions that are diffusing outward back in the opposite direction, from the outside toward the inside.  Guyton pg 57.  The potential change becomes great enough to block further net diffusion to the exterior despite the increase in the K+ ion concentration gradient.  In the normal large mammalian nerve fiber, the potential difference required is -94 mV with negativity inside the nerve fiber.

Define and discuss the Goldman equation for membrane potential:  When a membrane is permeable to several different ions, the diffusion potential that develops depend on 3 factors-- 1) the polarity of the electrical chare of each ion.  2) the permeability of the membrane to each ion, and  3) the concentrations of the respective ions on the inside and outside of the membrane.  Thus, the Goldman equation gives the calculated membrane potential on the inside of the membrane when 2 univalent positive ions Na+ and K+ and one univalent negative ion Cl- are involved.  

                C_Na+in P_Na+ + C_k+inP_k+ + C_Cl-outP_Cl-   
-61 log ___________________________________
                 C_Na+outP_Na+ + C_K+outP_K+ + C_Cl-inP_Cl-

Describe the mechanism of active transport, using the Na+, K+ ATPase system (electrogenic sodium pump) as your example.  What would happen to a cell if its sodium pump were completely disabled?:   Na+ is continually pumped to the outside of the cell and K+ to the inside.  It is an electrogenic pump because more positive charges area pumped to the outside than to the inside (3Na+ out : 2 K+ in) leaving a net deficit of positive ions on the inside as compared to the outside causing a negative charge inside the cell membrane.  If the pump ceased to work, then Na+ would build up in the inside to such a degree that the cell would burs (H2) follows Na+ out of the cell as it is pumped out).  ATP is used to pump out the Na+, once 2 K+ are attached to the transport protein on the outside and 3 Na+ are attached on the inside the energy generated by ATP causes the protein to pump out the Na+ and bring the K+ in.

Return to the MNA 2001 Homepage
Last Updated 09/06/01 08:53:13 PM